

Rectangular Tetrahedron
 |
 |
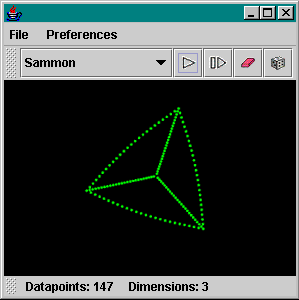 |
On the left the vertices of a rectangular tetrahedron (a slice off the corner of a cube) is shown projected into a plane using the Sammon map in HiSee. This tetrahedron has three fold rotational symmetry about the corner vertex. This symmetry is preserved in the projected image.
The edges connecting the vertices have been included in the two figures on the right. Three faces of the rectangular tetrahedron are isosceles right triangles and the remaining face is an equilateral triangle. The right triangle faces all share the corner vertex and their hypotenuses form the three edges of the equilateral triangle face. The angle between the hypotenuse and the leg of a right triangle is 45 degrees while the angle between any pair of sides of an equiangular triangle is 60 degrees.
It is impossible to preserve the angles of the faces when projecting the rectangular tetrahedron into a plane. PCA is a linear projection so that straight lines are mapped to straight lines. This is shown above in the center. The angles of the equilateral face are preserved but the right angles at the corner vertex have been stretched out to 120 degrees and the 45 degree angles have been compressed to 30 degrees.
The Sammon map of the rectangular tetrahedron is shown above on the right. The right angles at the corner vertices have been stretched out to 120 degrees just as with the PCA algorithm. The Sammon map is not linear and in the projected image the hypotenuses of the right triangles are slightly curved. This makes the angles between the hypotenuse and leg of a right triangle a little less than 45 degrees and the angles between the sides of the equiangular triangle a little more than 60 degrees. This provides a balance between the two types of triangles in the rectangular tetrahedron. This way we can see some aspects of the rectangular tetrahedron that are obscured when the vertices are simply connected with straight lines in the projected image.