HiSee

	A circle in a three dimensional space is a simple figure that is useful for illustrating HiSee's projection methods.
	A bouquet of circles is an arbitrary number of circles joined a single point. In a ten dimensional space five mutually perpendicular planes can meet at a single point.
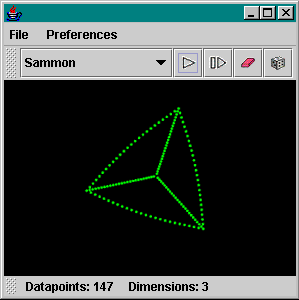	The projection of a tetrahedron shows that straight lines are turned into curves by the Sammon map as it tries to preserve the tetrahedron's geometry.
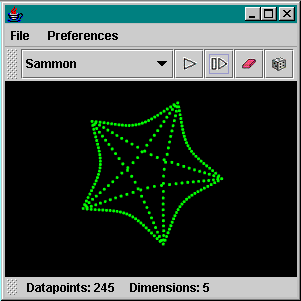	A regular 4-simplex is one of the simplest four dimensional objects and it is useful for illustrating the projection of objects whose dimension is too high for us to see directly. Other simplices are also considered.
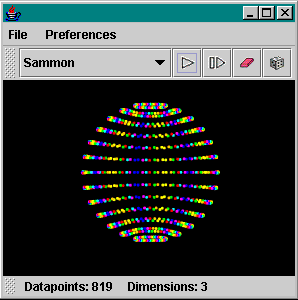	Projection of a sphere. Circles of latitude are shown as they often appear in map projections of the Earth. Circles of longitude are also evident by the colors of the points.
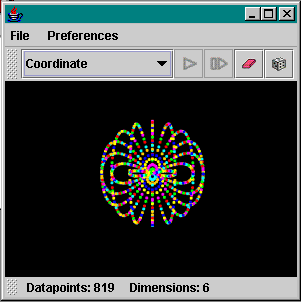	Like the Möbius strip the projective plane only has one side. However, unlike a Möbius strip it has no edges. It cannot sit in less than four dimensions without destroying its topology. But by viewing it under different projections using HiSee one can get a sense of its structure.